EXPANSIONES DE PI
Descubridor: Arquímedes (287-212 BC) averiguó que

PI = 3. 1415926535 8979323846 2643383279 5028841971 6939937510 5820974944 5923078164 0628620899 8628034825 3421170679 ...
La Fórmula de Vieta
2/PI =2/2 *
( 2 +
2 )/2 *
(2 + (
( 2 +
2) ) )/2 * ...c
La Fómula de Leibnitz
PI/4 = 1/1 - 1/3 + 1/5 - 1/7 + ...
El Producto de Wallis
PI/2 = 2/1 * 2/3 * 4/3 * 4/5 * 6/5 * 6/7 * ...2/PI = (1 - 1/2^2)(1 - 1/4^2)(1 - 1/6^2)...
La Fórmula de Lord Brouncker
4/PI = 1 + 1 ---------------- 2 + 3^2 ------------ 2 + 5^2 --------- 2 + 7^2 ...
(PI^2)/8 = 1/1^2 + 1/3^2 + 1/5^2 + ...
(PI^2)/24 = 1/2^2 + 1/4^2 + 1/6^2 + ...
(PI^2)/6 =(n = 1..
) 1/n^2 = 1/1^2 + 1/2^2 + 1/3^2 + ...
(o mas generalmente...)
(n = 1..
) 1/n^(2k) = (-1)^(k-1) PI^(2k) 2^(2k) B(2k) / ( 2(2k)!)
B(k) = el k th Número Bernoulli. ej. B0=1 B1=-1/2 B2=1/6 B4=-1/30 B6=1/42 B8=-1/30 B10=5/66. Números Bernoulli adicionales se definen como (n 0)B0 + (n 1)B1 + (n 2)B2 + ... + (n (n-1))B(N-1) = 0 presumiendo que todos los Números Impares de Bernoulli >1 son = 0. (n k) = coeficiente de binómico = n!/(k!(n-k)!)
e = 2.7182818284 5904523536 0287471352 6624977572 4709369995 9574966967 6277240766 3035354759 4571382178 5251664274 ...
e = lim (n -> 0) (1 + n)(1/n) ó e = lim (n ->
) (1 + 1/n)n
e =
(k=0..
) 1 / k!
gamma =
= 0.5772156649 0153286061 ...
= lim (n->
) ( 1 + 1/2 + 1/3 + 1/4 + ... + 1/n - ln(n) ) = 0.5772156649...
= -
e^-x ln x dx
i =
(-1)
i^2 = -1
1 / i = -i
i^(4k) = 1; i^(4k+1) = i; i^(4k+2) = -1; i^(4k+3) = -i (k = un entero)
( i ) =
(1/2)+
(1/2) i
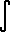


















No hay comentarios:
Publicar un comentario